The new generation of x-ray free-electron lasers opens up unique avenues for exploring matter under exotic and extreme conditions. Extensive spatial characterization of focused, typically (sub)micron-sized, laser beams is indispensable but, nevertheless, difficult to be accomplished due to excessive radiation intensities. Methods exist allowing indirect or semidirect focus characterization from a safe distance far from the focal point. Here we present a direct method of in-focus numerical phase recovery exploiting multishot desorption imprints in poly(methyl methacrylate). Shapes of the imprints serve as input data for the newly developed code PhaRe (phase recovery), inspired by the iterative Gerchberg-Saxton algorithm. A procedure of dynamic input-output mixing guarantees that the algorithm always converges to a self-consistent paraxial Helmholtz equation solution, which is thereafter optimized for transverse spatial coherence. Very good agreement with single-shot ablation imprints in lead tungstate (PbWO4) is found. The experiment is carried out at the Linac Coherent Light Source with a focused beam monochromatized at 800 eV. The results of the coherence optimization indicate that the act of monochromatization may have an effect on otherwise very good transverse coherence of free-electron laser beams.
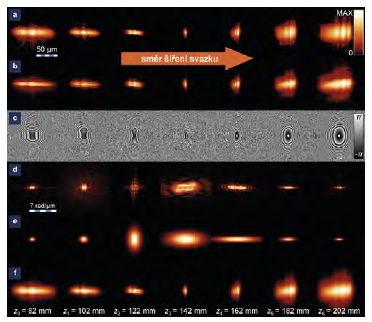
A visualization of input and output data generated by the PHAREcode. Row (a) depicts measured electrical field moduli, rows(b) and (c) show recovered (fully coherent and self-consistent) field amplitudes and phases modulo2π, rows (d) and (e) visualize therecoveredgSðκ;znÞfunction and the fit of the astigmatic Gaussian Schell model, and row (f) displays coherence optimized fieldamplitudes. All images, except for rows (d) and (e), are in the same scale, and each column is assigned a correspondingzposition.Images in rows (d) and (e) are in the reciprocal space and in the same scale. Both color scales for amplitude and phase are linear.